top of page
Classroom Interactions Teaching Experience
classroom-interactions-teaching-experience
Kanapaha Middle School | Pre-Algebra | Mrs. Litchfield | Grade 6 - 7
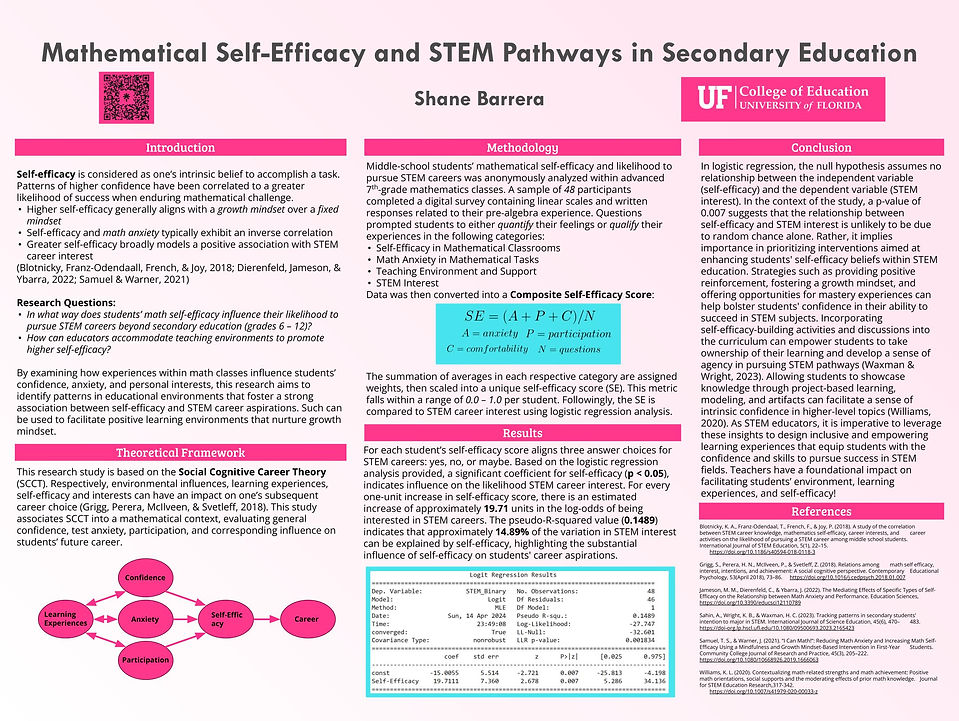
Overview: The purpose of this study is to investigate the correlation between middle-school students' self-efficacy scores in math and their likelihood to pursue STEM careers beyond secondary education (grades 6 - 12). By examining how experiences within math classes influence students' confidence levels, math anxiety, and personal interests, this research aims to identify patterns in educational environments that foster a strong association between self-efficacy and STEM career aspirations. The ultimate goal is to uncover insights that can inform the development of positive learning environments, enabling math teachers to effectively nurture student growth and enhance the likelihood of students pursuing careers in STEM fields.
The following research was presented at the UFTeach Symposium on Monday, April 22nd to fellow UFTeach students, mentor teachers and College of Education faculty:
Reading Journals:
-Week of 1/21/24: Observation Form 1
-Week of 1/28/24: Observation Form 2
-Week of 2/4/24: Journal Log 1
-Week of 2/11/24: Journal Log 2
-Week of 2/18/24: Journal Log 3
-Week of 2/25/24: Journal Log 4
-Week of 3/3/24: Journal Log 5
-Week of 3/17/24: Journal Log 6
-Week of 3/24/24: Journal Log 7
-Week of 3/31/24: Journal Log 8
-Week of 4/7/24: Journal Log 9
special-interest-research-project
Formative Assessments:
*Note: click on the formative assessment hyperlink to view
Overview: Throughout my experience working with 7th-grade pre-algebra students at Kanapaha Middle School, I implemented four types of formative assessments. These serve the purpose of diagnosing students' mathematical thinking, identifying sources of misconception, and adapting lesson plans to meet their needs. Each formative assessment possessed a specific purpose based on the relevant material per week.
Formative Assessment 1:
Students were provided with a sheet of paper to answer questions asking whether to Agree or Disagree on statements regarding linear equations. They were asked to justify their claims by explaining and drawing a visual. Generally, even if students did get all four questions correct, not they didn't always mention terms like "slope" or "y-intercept" in their explanations, prompting me to wonder about their understanding of these concepts. Additionally, some students appeared to have a slight misconception regarding "parallel" lines, possibly confusing horizontal parallels with parallels defined by equal slopes. This raised questions about the student's prior understanding of the term "parallel" and its application in linear equations. Finally, a student completed the assignment but inaccurately responded to two statements regarding systems of linear equations. Their misunderstanding seemed to stem from a confusion about the term "linear," suggesting a need for clarification or additional support. In summary, these assessments provided insights into students' understanding of linear equations and highlighted areas where further instruction or clarification may be necessary. Perhaps showing students varied examples of linear systems of equations (and not just the same universal illustration: positive linear slope about the origin) can prevent students from over-generalizing their interpretation of such. Often, students may extend their understanding of a linear system into a non-applicable scenario.
Formative Assessment 2:
Students were given a 5-Minute Quiz to independently solve for missing angles in a triangle using the triangle sum theorem. In one example, a student correctly defines a formula, identifies angles, and uses algebraic methods to solve for missing angles. They exhibit a step-by-step approach, following PEMDAS and combining like terms accurately. Another student, however, makes slight arithmetic errors and seems to struggle with understanding the concept of the triangle sum theorem. They incorrectly calculate angles by not accounting for the missing angle 'x' in the triangle. Similarly, a third student overlooks the missing angle 'x' and makes computational errors in algebraic evaluations, leading to decimal results. The first student demonstrates a strong understanding of algebraic procedures and the application of the triangle sum theorem. They exhibit meticulous calculation methods, which is crucial for avoiding simple computational errors. On the other hand, the second and third students seem to have difficulties grasping the concept of the triangle sum theorem. They may not fully understand the significance of the missing angle 'x' in the triangle and struggle with algebraic evaluations, leading to errors in their solutions. For the second and third students, I wonder about their approach to calculations. Do they rely heavily on calculators or mental math? Encouraging them to write out each step and double-check their work might help improve accuracy and reinforce understanding. In conclusion, these assessments reveal varying levels of understanding of the triangle sum theorem among students and highlight the importance of thorough calculation methods and conceptual understanding.
Formative Assessment 3:
Students were asked to provide a Muddiest Point, and Strongest Point regarding their understanding of the Pythagorean Theorem. The first student's response indicates a clear understanding of angle relationships and triangles but struggles with the Pythagorean Theorem (PT). This highlights a disconnect in bridging concepts from previous units to PT. The second student shows a strong understanding of angles of polygons but leaves the weakest point section blank. This suggests a need for clearer instructions or prompts to identify weaknesses. This student's approach suggests that visualizing word problems might be natural for some students but challenging for others. How can we support students with different learning styles? Lastly, the third student struggles with angle relationships of triangles but excels in understanding the Pythagorean Theorem. However, there's a misconception regarding the identification of legs and hypotenuse in a word problem. This student's approach highlights the importance of carefully reading and interpreting word problems to correctly identify the components of a geometric scenario. This formative assessment provided valuable insights into student perceptions and understanding. It underscores the importance of pacing lessons effectively and providing clear instructions for formative assessments. Perhaps showing a few real-world applications of the Pythagorean theorem can help students extend it beyond the generic right triangle examples they see within their text-books. Such often make students' understanding become procedural, leading them to make more errors by not paying attention to the geometric orientation of the right triangle itself.
Formative Assessment 4:
Students were asked to provide a 2-Minute Reflection on a module focused on statistics, specifically bi-variate data and scatterplots. Students' responses varied from feeling the unit was easy to having uncertainties about its practical application. These responses align with our prior knowledge of student perceptions and understanding of the lesson's content. The responses shed light on the importance of fostering students' interest and understanding of the practical applications of statistics. Adjusting the lesson to include more relevant and engaging examples could enhance student engagement and comprehension. Understanding why students categorize units as easy or difficult and addressing misconceptions about real-world applications of statistical concepts poses a challenge. How can we better communicate the relevance and importance of statistics in everyday life to students? This Formative Assessment provided insights into student perceptions and understanding of our own Scatter Plot Lesson presented as UF Teach educators. Understanding these trends can inform adjustments to future lessons to better meet students' needs and enhance engagement and comprehension.
bottom of page